yismxplusc: Geometric Proof of the Quadratic formula!

Proof of the quadratic formula:

Where did it come from?

The quadratic formula allows us to easily obtain the roots of any quadratic equation

a x 2 + b x + c = 0

. There is a very nice proof of this formula that uses geometry to give an intuitive understanding of this result. We show this proof below alongside the typical purely algebraic proof.

Theorem 1 (The Quadratic Formula): Let

a

b

, and

c

be real numbers with

a \neq 0

. Then the solutions to the quadratic equation

a x 2 + b x + c = 0

are

x = - b \pm b 2 - 4 a c - - - - - - - \sqrt 2 a

We require that $a \neq 0$ to ensure that $a x 2 + b x + c = 0$ is indeed a quadratic equation. If $a = 0$ then $a x 2 + b x + c = 0$ is the same as $b x + c = 0$ , which is a linear equation.

Proof (Geometric): Consider the quadratic equation $a x 2 + b x + c = 0$ with $a$ , $b$ , and $c$ as real numbers with $a \neq 0$ . Then:

(1)

a x 2 + b x + c a x 2 + b x x 2 + b a x = 0 = - c = - c a

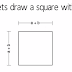
We illustrate the equation above as follows. We denote $x 2$ to be the area of square. Therefore, this square has side length $x$ .

We denote $b a x$ to be the area of a rectangle whose side lengths are $x$ and $b a$ .

Lastly, we denote $- c a$ to denote the area of a rectangle whose side lengths are unimportant.
Consider the rectangle $b a x$ . We separate this rectangle into two equal rectangles whose side lengths are $b 2 a$ and $x$ :
We take the first of these rectangles and attach the side with side length $x$ to the top of the square $x 2$ . WE take the second of these rectangles and attach the side with side length $x$ to the right side of the square $x 2$ . We add a smaller square with side length $b 2 a$ (and area $b 2 4 a 2$ to the lefthand side of the equation and we add the same amount of area to the righthand side of the equation to balance the equality: